Author(s): Peter Schorer
Fermat’s Last Theorem was originally stated by the 17th century mathematician Pierre de Fermat (1601-65). In the margin of an ancient Greek text on number theory that he was studying, he wrote: “There are no positive integers such that xn + yn = zn for n greater than 2. I’ve found a remarkable proof of this fact, but there is not enough space in the margin [of the text] to write it.”
Fermat’s Last Theorem was originally stated by the 17th century mathematician Pierre de Fermat (1601-65). In the margin of an ancient Greek text on number theory that he was studying, he wrote:
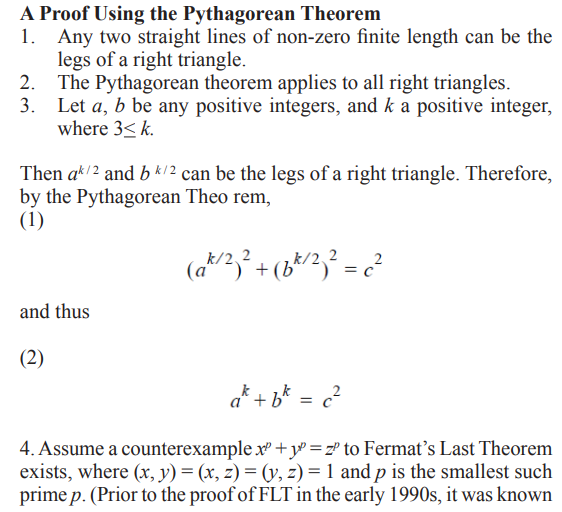
“There are no positive integers such that xn + yn = zn for n greater than 2. I’ve found a remarkable proof of this fact, but there is not enough space in the margin [of the text] to write it.”
For more than 300 years, no one was able to find a proof using the mathematical tools at Fermat’s disposal, or using any other, far more advanced, tools either, although the attempts produced numerous results, and at least one new branch of algebra, namely, ideal theory. Then in summer of 1993, a proof was announced by Princeton University mathematics professor Andrew Wiles. (Actually, Wiles announced a proof of a special case of the Shimura-Taniyama Conjecture — a special case that implies FLT.) Wiles’ proof was more than 100 pages long and had required more than seven years of dedicated effort. A gap in the proof was discovered later that summer, but Wiles, working with Richard Taylor, was able to fill it by the end of Sept. 1994.